Alessio and Tom work on the mathematical basis of string theory. The shapes we work with can be classified into three different kinds: Fano (positively curved), Calabi–Yau (flat) and General Type (negatively curved). Fano and Calabi–Yau shapes play a special role in geometry as “atomic pieces” when breaking complex shapes down to simpler ones, and in physics as backgrounds for string theory. String theory requires spacetime to have ten dimensions. The extra six dimension are curled up in what we call “inner space”. Fano and Calabi-Yau shapes are possible shapes of this inner space. Alessio and Tom aim to classify and write down the equations of these Fano and Calabi-Yau atomic pieces thereby producing a vast “periodic table” of all possible shapes and we have made many collaborative drawings exploring possible classifications.

String theory is a leading candidate for a “theory of everything”. It postulates that the fundamental objects in physics are not point-like particles but strings. These strings move in a background that, in addition to space and time, has extra hidden dimensions curled up in (depending on the version of the theory) either a 3-dimensional Fano or Calabi-Yau shape (3 complex dimensions = 6 real dimensions (there are three tiny dimensions ‘curled up’ inside three larger dimensions). Thus, our classification of shapes is also a directory of all the possible background geometries of string theory. There is no image of the background geometry of string theory and we have worked together to experiment with what this might look like.

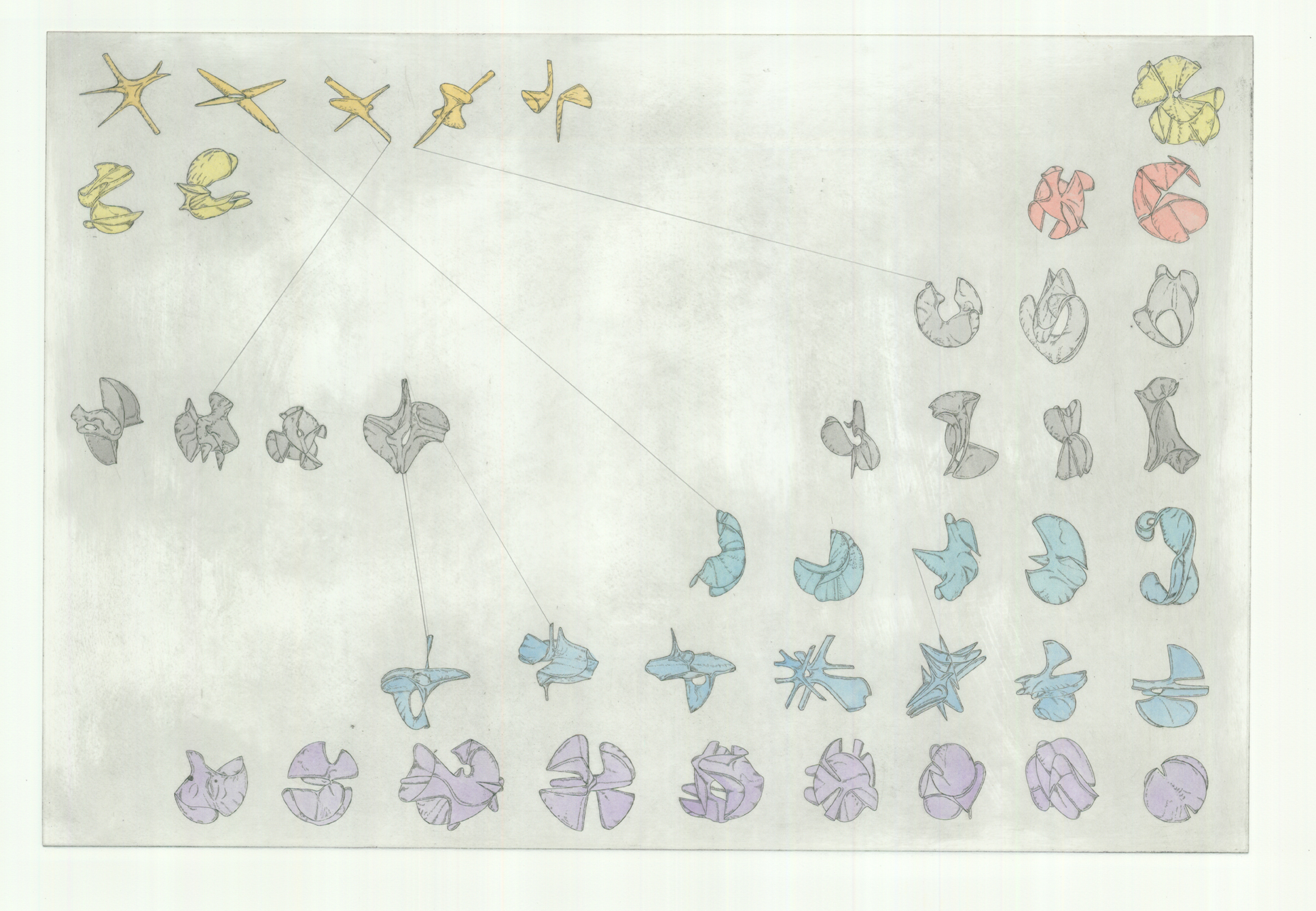
To tackle our classification, we will show how to associate a Fano shape to every reflexive polytope. A reflexive polytope is a geometric object closely related to the “platonic solids” that we learn about in school. To give an idea of the size and complexity of the problem, the complete list of 4-dimensional reflexive polytopes is known and there are nearly half a billion of these. We have unfolded these 4-dimensional reflexive polytopes, modelled them in 3 dimensions and created a toolkit for others to do the same.

In string theory, it can happen that two mathematically very different background geometries produce the same physics. When this happens, the two backgrounds are said to be “mirror” to each other. Mirror symmetry is a most fascinating aspect of string theory, and one of our key aims is to give the first truly transparent explanation of this phenomenon. The idea of the mirror, the act of turning inside out and ubiquity as image and metaphor in relation to the fourth dimension are important themes in our work together.

We are inspired when we see the same structures arise independently and for separate reasons in different parts of mathematics and science — this reveals deep connections between seemingly unrelated scientific disciplines. An example was the discovery of group theory in mathematics and in quantum physics at the turn of the 20th century. For example Calabi-Yau shapes arise independently in geometry and in string theory; cluster algebras are found at the same time in algebra and in the geometry of mirror symmetry.

We began working together on the 18/03/2011 and we have worked together on these shapes ever since. The initial interest I had was on extracting the shapes from computers, drawing, etching and modelling them and experimenting with approaches to classifying them. Later we became interested in the informal role of drawing in mathematics and wrote a series of articles together. In 2012, while working on a practice based PhD I was also Leverhulme artist in residence in the maths department at Imperial College and began collaborating with mathematicians who worked on knot topologies. These collaborations have been in parallel with my work investigating biological forms and process and has often intersected and overlapped. For example in our publications on the Four dimensional tree and The Origami Embryo as a Space Time Worm (In Drawing Processes of Life, Forthcoming in 2023) We continued to work together by writing our collaboration into Alessio and Tom’s EPSRC and ERC grants. Drawing and visualising in the fourth spatial dimension has been central to our collaboration from the onset and now after eleven years we are making the first physical models of 4d irregular polytopes and the inner spaces of string theory and very much looking forward to sharing more of this new work with you soon.